Are you ready to find 'how to write discrete math proofs'? You can find all the material on this web page.
The ProcessFind a impervious to practice. You can find the best practice proofs in the important text of letter a textbook that’s in writing at your even. ...Brainstorm. For some moderately difficult impervious, it’s unlikely that you’ll be fit to start with the premise and proceed step aside step until you reach the conclusion.Write a draft. ...Fill in the inside information. ...
Table of contents
- How to write discrete math proofs in 2021
- Proof techniques in discrete mathematics pdf
- Direct proof examples discrete math
- Direct proof examples and solutions
- Discrete math proofs cheat sheet
- Discrete mathematics example problems
- Direct proof examples and solutions pdf
- Discrete math proof solver
How to write discrete math proofs in 2021
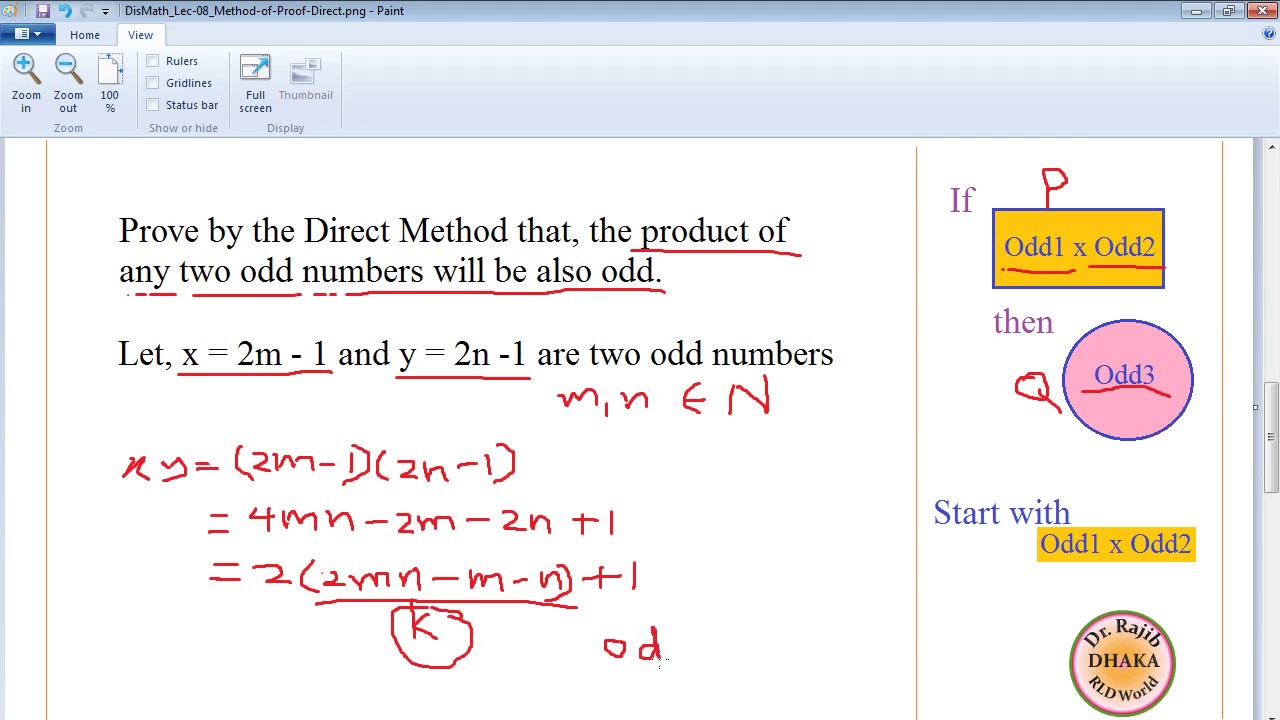 This image shows how to write discrete math proofs.
This image shows how to write discrete math proofs.
Proof techniques in discrete mathematics pdf
 This picture representes Proof techniques in discrete mathematics pdf.
This picture representes Proof techniques in discrete mathematics pdf.
Direct proof examples discrete math
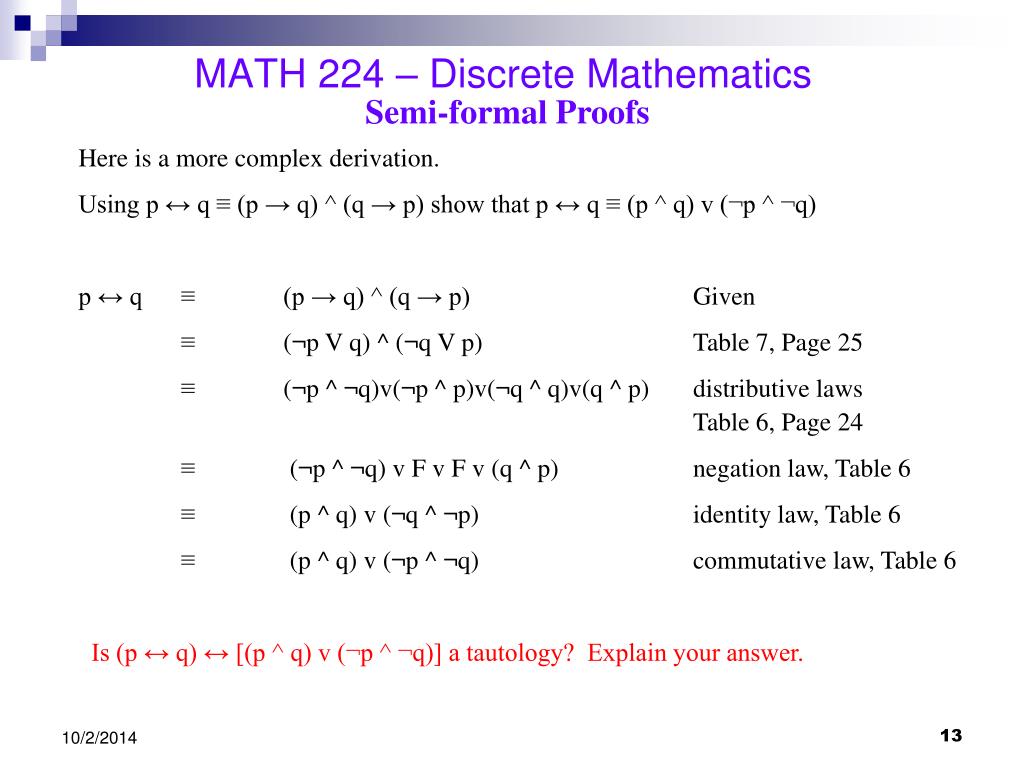 This picture representes Direct proof examples discrete math.
This picture representes Direct proof examples discrete math.
Direct proof examples and solutions
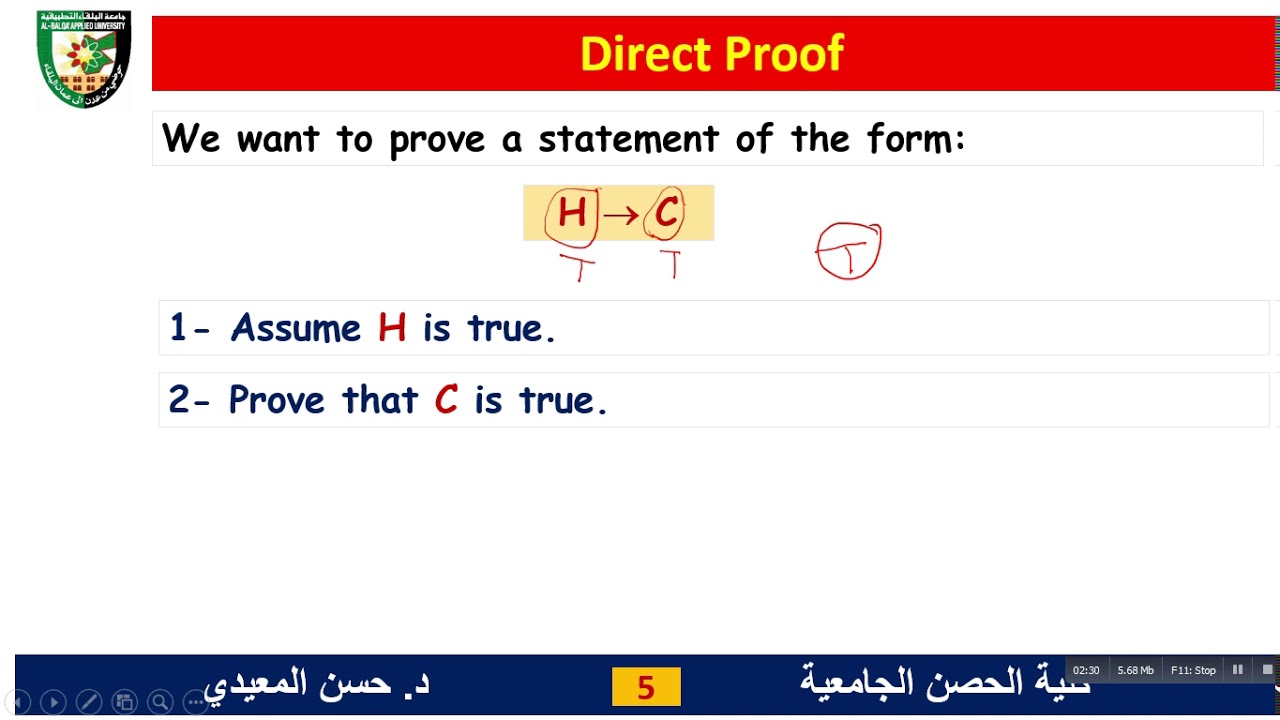 This image illustrates Direct proof examples and solutions.
This image illustrates Direct proof examples and solutions.
Discrete math proofs cheat sheet
 This picture illustrates Discrete math proofs cheat sheet.
This picture illustrates Discrete math proofs cheat sheet.
Discrete mathematics example problems
 This image representes Discrete mathematics example problems.
This image representes Discrete mathematics example problems.
Direct proof examples and solutions pdf
 This picture representes Direct proof examples and solutions pdf.
This picture representes Direct proof examples and solutions pdf.
Discrete math proof solver
 This picture demonstrates Discrete math proof solver.
This picture demonstrates Discrete math proof solver.
Is it possible to write a proof of a statement?
Anyone who doesn't believe there is creativity in mathematics clearly has not tried to write proofs. Finding a way to convince the world that a particular statement is necessarily true is a mighty undertaking and can often be quite challenging. There is not a guaranteed path to success in the search for proofs.
Which is an example of a mathematical proof?
Example: The question tells you to “Prove that if x is a non-zero element of R, then x has a multiplicative inverse.” Your proof should be formatted something like this: If x is a non-zero element of R, then x has a multiplicative inverse. Pf: [Insert proof here].
What is the beginning and middle of a proof?
A proof is a clear and well written argument, and just like a story, it has a beginning, middle, and end. The beginning of your proof asserts or assumes what we know to be true using definition and theorems. The middle of the proof are statements that follow logically from preceding statements.
How does a direct proof begin and end?
A direct proof begins with an assertion and will end with the statement of what is trying to be proved. So how do we go about constructing a proof? A proof is a clear and well written argument, and just like a story, it has a beginning, middle, and end.
Last Update: Oct 2021